Question:
How is the erosive shear stress τ at a certain point influenced by the neighboring bed geometries or the neighboring bed roughness?
Pictures:
Difficulty:
This is a typical Inverse Problem! As potentially any point in the model affects the shear stress even over longer distances due to its ability to alter the water level with its properties ZF=Bottom Elevation or KS=Equivalent Sand Roughness.
Experimental Setup:
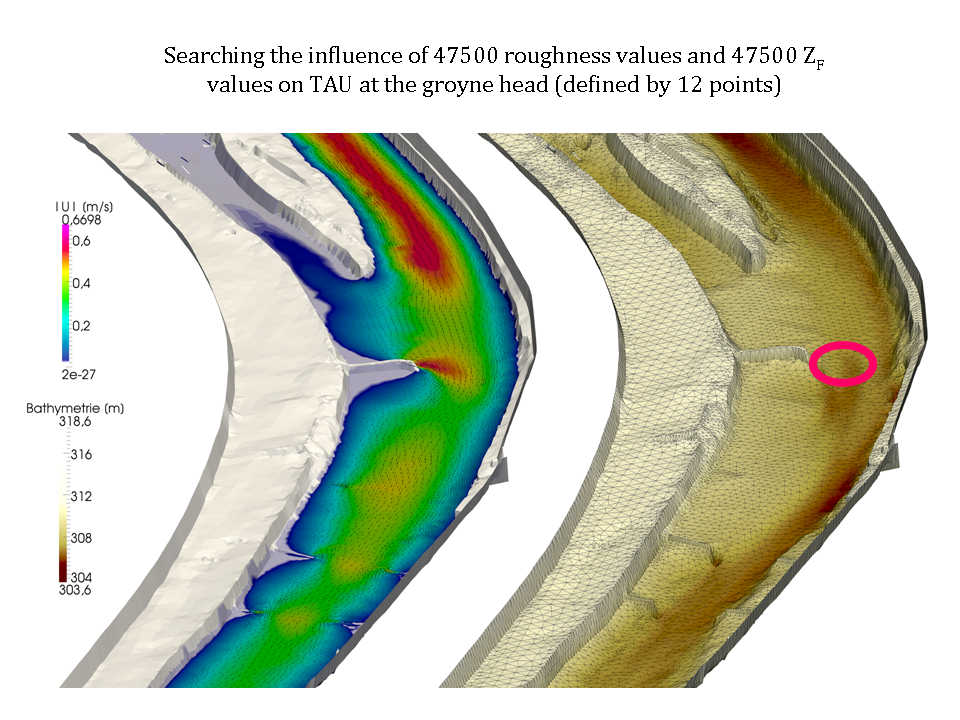
A part of the Danube (official Telemac-2D validation case 221_donau) is shown in the following picture.
- The purple ring marks 12 mesh nodes which are right in the middle of the groyne head scour.
- 47500 points are examined to quantify their influence through ZF=Bottom Elevation or KS=Equivalent Sand Roughness
- The model runs until the adjoints are quasi steady ~ 4500s
Interpretation of the resulting adjoints as a map:
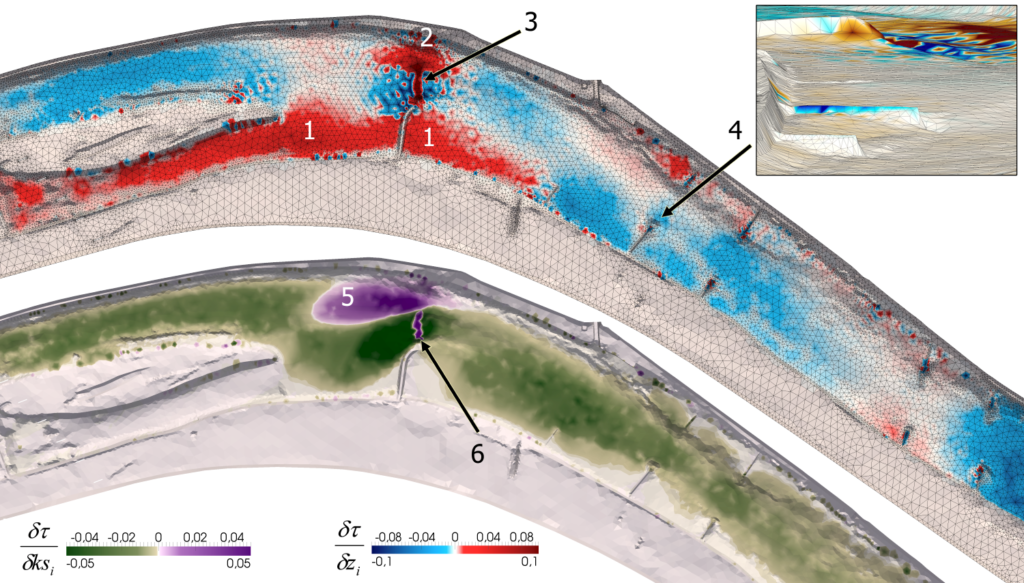
Bathymetrie
The dependency of the bathymetry on τ at the simulated flow conditions is dominated by the obvious separation of the 2 arms around the upstream island. If the southern arm, which has low flow, is lifted, then more water is pushed to the main channel (1). The same happens if the surrounding of the groin and the opposite site (2) of the cross section are elevated. A kind of funnel effect increases the shear stress. Decreasing the scour itself increases the shear stress as well (3).
The perspective view from downstream (4) reveals that some other groins have a high impact, as they influence with their back draft the water level in the examined area. The lower groins, which are smaller and shaded by the bigger ones therefore don’t influence the examination zone anymore. Again the reader shall be warned that only a change of few decimeters in any topographic feature might change the result totally. The general noise in the adjoints origins from bumps and holes in the bathymetry. Following the direction of the adjoints will smoothen the main channel and therefore accelerate flow and increase the shear stress.
Roughness
On the contrary for bed roughness a clear tendency is visible: the smoother the bed along the main channel, the higher the shear stress at the groin head scour. If the roughness gets higher on the opposite shore (5), more water is pressed to the scour. Classic methods (numerical and physical) would have given a rough idea about this dependency, but for the first time a hydraulic model can exactly define the spatial limits of the relevant area.The scour itself has a different tendency (6): Increasing the roughness in the target point itself obviously increases the local shear stress.
DISCLAIMER: Adjoints computed by TELEMAC-2D-AD describe the change of the output τ as a linear relation of its specific input. Therefore extrapolations for other input parameter sets ma be wrong!
Download and experiment with the trail show case:
The example is available for Download as ZIP: -> Link (Registered Users Only!)
- The executable is compiled for Ubuntu 64bit.
- Unpack the folder
- Open a Teminal in this folder and execute „./Danube-Adj-tau_vs_zf+ks.exe“
- Results will appear in the file „T2DRES“ in the same folder
If your not already registered: Contact us!: